| ROCKET PROPULSION |
- Thrust
- Conservation of Momentum
- Impulse & Momentum
- Combustion & Exhaust Velocity
- Specific Impulse
- Rocket Engines
- Power Cycles
- Engine Cooling
- Solid Rocket Motors
- Monopropellant Engines
- Staging
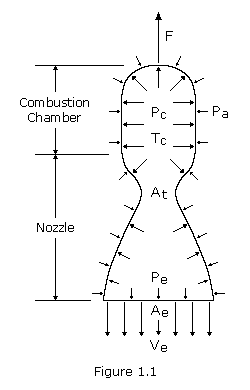
Thrust is the force that propels a rocket or spacecraft and is
measured in pounds, kilograms or Newtons. Physically speaking, it is the
result of pressure which is exerted on the wall of the combustion
chamber.
Figure 1.1 shows a combustion chamber with an opening, the
nozzle, through which gas can escape. The pressure distribution within
the chamber is asymmetric; that is, inside the chamber the pressure
varies little, but near the nozzle it decreases somewhat. The force due
to gas pressure on the bottom of the chamber is not compensated for from
the outside. The resultant force F due to the internal and
external pressure difference, the thrust, is opposite to the direction
of the gas jet. It pushes the chamber upwards.
To create high speed exhaust gases, the necessary high
temperatures and pressures of combustion are obtained by using a very
energetic fuel and by having the molecular weight of the exhaust gases
as low as possible. It is also necessary to reduce the pressure of the
gas as much as possible inside the nozzle by creating a large section
ratio. The section ratio, or expansion ratio, is defined as the area of
the exit Ae divided by the area of the throat At.
The thrust F is the resultant of the forces due to the
pressures exerted on the inner and outer walls by the combustion gases
and the surrounding atmosphere, taking the boundary between the inner
and outer surfaces as the cross section of the exit of the nozzle. As we
shall see in the next section, applying the principle of the
conservation of momentum gives
where q is the rate of the ejected mass flow, Pa the pressure of the ambient atmosphere, Pe the pressure of the exhaust gases and Ve their ejection speed. Thrust is specified either at sea level or in a vacuum.
The linear momentum (p), or simply momentum, of a particle is the product of its mass and its velocity. That is,
Newton expressed his second law of motion in terms of momentum, which
can be stated as "the resultant of the forces acting on a particle is
equal to the rate of change of the linear momentum of the particle". In
symbolic form this becomes
which is equivalent to the expression F=ma.
If we have a system of particles, the total momentum P of
the system is the sum of the momenta of the individual particles. When
the resultant external force acting on a system is zero, the total
linear momentum of the system remains constant. This is called the
principle of conservation of linear momentum. Let's now see how this principle is applied to rocket mechanics.
Consider a rocket drifting in gravity free space. The rocket's engine is fired for time 
t
and, during this period, ejects gases at a constant rate and at a
constant speed relative to the rocket (exhaust velocity). Assume there
are no external forces, such as gravity or air resistance.
t
and, during this period, ejects gases at a constant rate and at a
constant speed relative to the rocket (exhaust velocity). Assume there
are no external forces, such as gravity or air resistance.
Figure 1.2(a) shows the situation at time t. The rocket and fuel have a total mass M and the combination is moving with velocity v as seen from a particular frame of reference. At a time t later the configuration has changed to that shown in Figure 1.2(b). A mass M has been ejected from the rocket and is moving with velocity u as seen by the observer. The rocket is reduced to mass M-M and the velocity v of the rocket is changed to v+v.
t later the configuration has changed to that shown in Figure 1.2(b). A mass M has been ejected from the rocket and is moving with velocity u as seen by the observer. The rocket is reduced to mass M-M and the velocity v of the rocket is changed to v+v.
Because there are no external forces, dP/dt=0. We can write, for the time interval t
t
where P2 is the final system momentum, Figure 1.2(b), and P1 is the initial system momentum, Figure 1.2(a). We write
If we let t approach zero, v/t approaches dv/dt, the acceleration of the body. The quantity M is the mass ejected in t; this leads to a decrease in the mass M of the original body. Since dM/dt, the change in mass of the body with time, is negative in this case, in the limit the quantity M/t is replaced by -dM/dt. The quantity u-(v+v) is Vrel, the relative velocity of the ejected mass with respect to the rocket. With these changes, equation (1.4) can be written as
t approach zero, v/t approaches dv/dt, the acceleration of the body. The quantity M is the mass ejected in t; this leads to a decrease in the mass M of the original body. Since dM/dt, the change in mass of the body with time, is negative in this case, in the limit the quantity M/t is replaced by -dM/dt. The quantity u-(v+v) is Vrel, the relative velocity of the ejected mass with respect to the rocket. With these changes, equation (1.4) can be written as
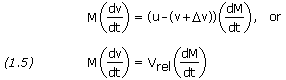
The right-hand term depends on the characteristics of the rocket and,
like the left-hand term, has the dimensions of a force. This force is
called the thrust, and is the reaction force exerted on the
rocket by the mass that leaves it. The rocket designer can make the
thrust as large as possible by designing the rocket to eject mass as
rapidly as possible (dM/dt large) and with the highest possible relative speed (Vrel large).
In rocketry, the basic thrust equation is written as
where q is the rate of the ejected mass flow, Ve is the exhaust gas ejection speed, Pe is the pressure of the exhaust gases at the nozzle exit, Pa is the pressure of the ambient atmosphere, and Ae is the area of the nozzle exit. The product qVe, which we derived above (Vrel × dM/dt), is called the momentum, or velocity, thrust. The product (Pe-Pa)Ae,
called the pressure thrust, is the result of unbalanced pressure forces
at the nozzle exit. As we shall see latter, maximum thrust occurs when Pe=Pa.
Click here for example problem #1.1
(use your browser's "back" function to return)
Equation (1.6) may be simplified by the definition of an effective exhaust gas velocity, C, defined as
Equation (1.6) then reduces to
In the preceding section we saw that Newton's second law may be expressed in the form
Multiplying both sides by dt and integrating from a time t1 to a time t2, we write
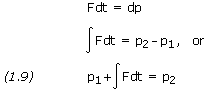
The integral is a vector known as the linear impulse, or simply the impulse, of the force F during the time interval considered. The equation expresses that, when a particle is acted upon by a force F during a given time interval, the final momentum p2 of the particle may be obtained by adding its initial momentum p1 and the impulse of the force F during the interval of time.
When several forces act on a particle, the impulse of each of the
forces must be considered. When a problem involves a system of
particles, we may add vectorially the momenta of all the particles and
the impulses of all the forces involved. When can then write
For a time interval t, we may write equation (1.10) in the form
t, we may write equation (1.10) in the form
Let us now see how we can apply the principle of impulse and momentum to rocket mechanics.
Consider a rocket of initial mass M which it launched vertically at time t=0. The fuel is consumed at a constant rate q and is expelled at a constant speed Ve relative to the rocket. At time t, the mass of the rocket shell and remaining fuel is M-qt, and the velocity is v. During the time interval t, a mass of fuel qt is expelled. Denoting by u the absolute velocity of the expelled fuel, we apply the principle of impulse and momentum between time t and time t+t. Please note, this derivation neglects the effect of air resistance.
t, a mass of fuel qt is expelled. Denoting by u the absolute velocity of the expelled fuel, we apply the principle of impulse and momentum between time t and time t+t. Please note, this derivation neglects the effect of air resistance.
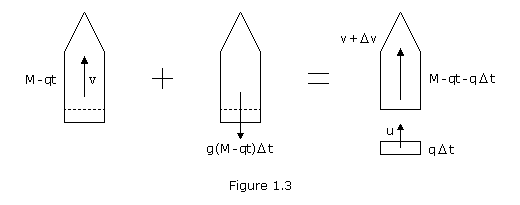
We write
We divide through by t and replace u-(v+v) with Ve, the velocity of the expelled mass relative to the rocket. As t approaches zero, we obtain
t and replace u-(v+v) with Ve, the velocity of the expelled mass relative to the rocket. As t approaches zero, we obtain
Separating variables and integrating from t=0, v=0 to t=t, v=v, we obtain
which equals
The term -gt in equation (1.15) is the result of Earth's gravity pulling on the rocket. For a rocket drifting in space, -gt
is not applicable and can be omitted. Furthermore, it is more
appropriate to express the resulting velocity as a change in velocity,
or V. Equation (1.15) thus becomes
V. Equation (1.15) thus becomes
Note that M represents the initial mass of the rocket and M-qt the final mass. Therefore, equation (1.16) is often written as
where mo/mf is called the mass ratio.
Equation (1.17) is also known as Tsiolkovsky's rocket equation, named
after Russian rocket pioneer Konstantin E. Tsiolkovsky (1857-1935) who
first derived it.
In practical application, the variable Ve is usually replaced by the effective exhaust gas velocity, C. Equation (1.17) therefore becomes
Alternatively, we can write
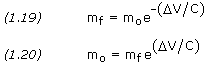
where e is a mathematical constant approximately equal to 2.71828.
For many spacecraft maneuvers it is necessary to calculate the
duration of an engine burn required to achieve a specific change in
velocity. Rearranging variables, we have
The combustion process involves the oxidation of constituents in the
fuel that are capable of being oxidized, and can therefore be
represented by a chemical equation. During a combustion process the mass
of each element remains the same. Consider the reaction of methane with
oxygen
This equation states that one mole of methane reacts with two moles
of oxygen to form one mole of carbon dioxide and two moles of water.
This also means that 16 g of methane react with 64 g of oxygen to form
44 g of carbon dioxide and 36 g of water. All the initial substances
that undergo the combustion process are called the reactants, and the substances that result from the combustion process are called the products.
The above combustion reaction is an example of a stoichiometric mixture,
that is, there is just enough oxygen present to chemically react with
all the fuel. The highest flame temperature is achieved under these
conditions, however it is often desirable to operate a rocket engine at a
"fuel-rich" mixture ratio. Mixture ratio is defined as the mass flow of oxidizer divided by the mass flow of fuel.
Consider the following reaction of kerosene(1) with oxygen,
Given the molecular weight of C12H26 is 170 and that of O2 is 32, we have a mixture ratio of
which is typical of many rocket engines using kerosene, or RP-1, fuel.
The optimum mixture ratio is typically that which will deliver the highest engine performance (measured by specific impulse),
however in some situations a different O/F ratio results in a better
overall system. For a volume-constrained vehicle with a low-density fuel
such as liquid hydrogen, significant reductions in vehicle size can be
achieved by shifting to a higher O/F ratio. In that case, the losses in
performance are more than compensated for by the reduced fuel tankage
requirement. Also consider the example of bipropellant systems using
NTO/MMH, where a mixture ratio of 1.67 results in fuel and oxidizer
tanks of equal size. Equal sizing simplifies tank manufacturing, system
packaging, and integration.
As we have seen previously, impulse thrust is equal to the
product of the propellant mass flow rate and the exhaust gas ejection
speed. The ideal exhaust velocity is given by
where k is the specific heat ratio, R* is the universal gas constant (8,314.4621 J/kmol-K in SI units, or 49,720 ft-lb/(slug-mol)-oR in U.S. units), Tc is the combustion temperature, M is the average molecular weight of the exhaust gases, Pc is the combustion chamber pressure, and Pe is the pressure at the nozzle exit.
Specific heat ratio(2) varies depending on the
composition and temperature of the exhaust gases, but it is usually
about 1.2. The thermodynamics involved in calculating combustion
temperatures are quite complicated, however, flame temperatures
generally range from about 2,500 to 3,600 oC (4,500-6,500 oF). Chamber pressures can range from about about 7 to 250 atmospheres. Pe should be equal to the ambient pressure at which the engine will operate, more on this later.
From equation (1.22) we see that high chamber temperature and
pressure, and low exhaust gas molecular weight results in high ejection
velocity, thus high thrust. Based on this criterion, we can see why
liquid hydrogen is very desirable as a rocket fuel.
It should be pointed out that in the combustion process there will be
a dissociation of molecules among the products. That is, the high heat
of combustion causes the separation of molecules into simpler
constituents that are then capable of recombining. Consider the reaction
of kerosene with oxygen. The true products of combustion will be an
equilibrium mixture of atoms and molecules consisting of C, CO, CO2, H, H2, H2O, HO, O, and O2. Dissociation has a significant effect on flame temperature.
If you wish to learn more about the thermodynamics of rockets engines, please consider reading the appendix Rocket Thermodynamics.
Or you can skip all the science and just look up the numbers you need. See Propellant Combustion Charts
to find optimum mixture ratio, adiabatic flame temperature, gas
molecular weight, and specific heat ratio for some common rocket
propellants.
(1) In dealing with combustion of liquid hydrocarbon fuels it is
convenient to express the composition in terms of a single hydrocarbon,
even though it is a mixture of many hydrocarbons. Thus gasoline is
usually considered to be octane, C8H18, and kerosene is considered to be dodecane, C12H26.
(2) Specific heat, or heat capacity,
represents the amount of heat necessary to raise the temperature of one
gram of a substance one degree C. Specific heat is measured at
constant-pressure, CP, or at constant-volume, CV. The ratio CP/CV is called the specific heat ratio, represented by k or  .
|
The specific impulse of a rocket, Isp, is the ratio of the thrust to the flow rate of the weight ejected, that is
where F is thrust, q is the rate of mass flow, and go is standard gravity (9.80665 m/s2).
Specific impulse is expressed in seconds. When the thrust and the
flow rate remain constant throughout the burning of the propellant, the
specific impulse is the time for which the rocket engine provides a
thrust equal to the weight of the propellant consumed.
For a given engine, the specific impulse has different values on
the ground and in the vacuum of space because the ambient pressure is
involved in the expression for the thrust. It is therefore important to
state whether specific impulse is the value at sea level or in a vacuum.
There are a number of losses within a rocket engine, the main
ones being related to the inefficiency of the chemical reaction
(combustion) process, losses due to the nozzle, and losses due to the
pumps. Overall, the losses affect the efficiency of the specific
impulse. This is the ratio of the real specific impulse (at sea level,
or in a vacuum) and the theoretical specific impulse obtained with an
ideal nozzle from gases coming from a complete chemical reaction.
Calculated values of specific impulse are several percent higher than
those attained in practice.
From Equation (1.8) we can substitute qC for F in Equation (1.23), thus obtaining
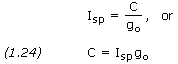
Equation (1.24) is very useful when solving Equations (1.18) through (1.21). It is rare we are given the value of C directly, however rocket engine specific impulse is a commonly given parameter from which we can easily calculate C.
Another important figure of merit for evaluating rocket performance is the characteristic exhaust velocity, C* (pronounced "C star"), which is a measure of the energy available from the combustion process and is given by
where Pc is the combustion chamber pressure and At is the area of the nozzle throat. Delivered values of C* range from about 1,333 m/s for monopropellant hydrazine up to about 2,360 m/s for cryogenic oxygen/hydrogen.
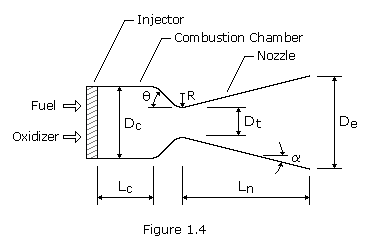
A typical rocket engine consists of the nozzle, the combustion chamber,
and the injector, as shown in Figure 1.4. The combustion chamber is
where the burning of propellants takes place at high pressure. The
chamber must be strong enough to contain the high pressure generated by,
and the high temperature resulting from, the combustion process.
Because of the high temperature and heat transfer, the chamber and
nozzle are usually cooled. The chamber must also be of sufficient length
to ensure complete combustion before the gases enter the nozzle.
Nozzle
The function of the nozzle is to convert the chemical-thermal
energy generated in the combustion chamber into kinetic energy. The
nozzle converts the slow moving, high pressure, high temperature gas in
the combustion chamber into high velocity gas of lower pressure and
temperature. Since thrust is the product of mass and velocity, a very
high gas velocity is desirable. Nozzles consist of a convergent and
divergent section. The minimum flow area between the convergent and
divergent section is called the nozzle throat. The flow area at the end
of the divergent section is called the nozzle exit area. The nozzle is
usually made long enough (or the exit area is great enough) such that
the pressure in the combustion chamber is reduced at the nozzle exit to
the pressure existing outside the nozzle. It is under this condition, Pe=Pa where Pe is the pressure at the nozzle exit and Pa
is the outside ambient pressure, that thrust is maximum and the nozzle
is said to be adapted, also called optimum or correct expansion. When Pe is greater than Pa, the nozzle is under-extended. When the opposite is true, it is over-extended.
We see therefore, a nozzle is designed for the altitude at which
it has to operate. At the Earth's surface, at the atmospheric pressure
of sea level (0.1 MPa or 14.7 psi), the discharge of the exhaust gases
is limited by the separation of the jet from the nozzle wall. In the
cosmic vacuum, this physical limitation does not exist. Therefore, there
have to be two different types of engines and nozzles, those which
propel the first stage of the launch vehicle through the atmosphere, and
those which propel subsequent stages or control the orientation of the
spacecraft in the vacuum of space.
The nozzle throat area, At, can be found if the
total propellant flow rate is known and the propellants and operating
conditions have been selected. Assuming perfect gas law theory, we have
where q is the propellant mass flow rate, Pt is the gas pressure at the nozzle throat, Tt is the gas temperature at the nozzle throat, R* is the universal gas constant, and k is the specific heat ratio. Pt and Tt are given by
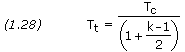
where Pc is the combustion chamber pressure and Tc is the combustion chamber flame temperature.
The hot gases must be expanded in the diverging section of the nozzle
to obtain maximum thrust. The pressure of these gases will decrease as
energy is used to accelerate the gas. We must find that area of the
nozzle where the gas pressure is equal to the outside atmospheric
pressure. This area will then be the nozzle exit area.
Mach number Nm is the ratio of the gas velocity
to the local speed of sound. The Mach number at the nozzle exit is
given by the perfect gas expansion expression
where Pa is the pressure of the ambient atmosphere.
The nozzle exit area, Ae, corresponding to the exit Mach number is given by
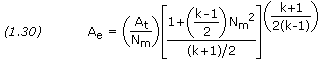
The section ratio, or expansion ratio, is defined as the area of the exit Ae divided by the area of the throat At.
For launch vehicles (particularly first stages) where the ambient
pressure varies during the burn period, trajectory computations are
performed to determine the optimum exit pressure. However, an additional
constraint is the maximum allowable diameter for the nozzle exit cone,
which in some cases is the limiting constraint. This is especially true
on stages other than the first, where the nozzle diameter may not be
larger than the outer diameter of the stage below. For space engines,
where the ambient pressure is zero, thrust always increases as nozzle
expansion ratio increases. On these engines, the nozzle expansion ratio
is generally increased until the additional weight of the longer nozzle
costs more performance than the extra thrust it generates.
(For additional information, please see Supplement #1: Optimizing Expansion for Maximum Thrust.)
Since the flow velocity of the gases in the converging section of
the rocket nozzle is relatively low, any smooth and well-rounded
convergent nozzle section will have very low energy loses. By contrast,
the contour of the diverging nozzle section is very important to
performance, because of the very high flow velocities involved. The
selection of an optimum nozzle shape for a given expansion ratio is
generally influenced by the following design considerations and goals:
(1) uniform, parallel, axial gas flow at the nozzle exit for maximum
momentum vector, (2) minimum separation and turbulence losses within the
nozzle, (3) shortest possible nozzle length for minimum space envelope,
weight, wall friction losses, and cooling requirements, and (4) ease of
manufacturing.
Conical nozzle: In early rocket engine
applications, the conical nozzle, which proved satisfactory in most
respects, was used almost exclusively. A conical nozzle allows ease of
manufacture and flexibility in converting an existing design to higher
or lower expansion ratio without major redesign.
The configuration of a typical conical nozzle is shown in Figure
1.4. The nozzle throat section has the contour of a circular arc with
radius R, ranging from 0.25 to 0.75 times the throat diameter, Dt. The half-angle of the nozzle convergent cone section, 
, can range from 20 to 45 degrees. The divergent cone half-angle, 
,
varies from approximately 12 to 18 degrees. The conical nozzle with a
15-degree divergent half-angle has become almost a standard because it
is a good compromise on the basis of weight, length, and performance.
, can range from 20 to 45 degrees. The divergent cone half-angl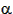e, ,
varies from approximately 12 to 18 degrees. The conical nozzle with a
15-degree divergent half-angle has become almost a standard because it
is a good compromise on the basis of weight, length, and performance.
Since certain performance losses occur in a conical nozzle as a
result of the nonaxial component of the exhaust gas velocity, a
correction factor,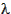 
,
is applied in the calculation of the exit-gas momentum. This factor
(thrust efficiency) is the ratio between the exit-gas momentum of the
conical nozzle and that of an ideal nozzle with uniform, parallel, axial
gas-flow. The value of can be expressed by the following equation:
,
is applied in the calculation of the exit-gas momentum. This factor
(thrust efficiency) is the ratio between the exit-gas momentum of the
conical nozzle and that of an ideal nozzle with uniform, parallel, axial
gas-flow. The value of 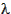can be expressed by the following equation:
Bell nozzle: To gain higher performance and shorter
length, engineers developed the bell-shaped nozzle. It employs a
fast-expansion (radial-flow) section in the initial divergent region,
which leads to a uniform, axially directed flow at the nozzle exit. The
wall contour is changed gradually enough to prevent oblique shocks.
An equivalent 15-degree half-angle conical nozzle is commonly
used as a standard to specify bell nozzles. For instance, the length of
an 80% bell nozzle (distance between throat and exit plane) is 80% of
that of a 15-degree half-angle conical nozzle having the same throat
area, radius below the throat, and area expansion ratio. Bell nozzle
lengths beyond approximately 80% do not significantly contribute to
performance, especially when weight penalties are considered. However,
bell nozzle lengths up to 100% can be optimum for applications stressing
very high performance.
One convenient way of designing a near optimum thrust bell nozzle
contour uses the parabolic approximation procedures suggested by G.V.R.
Rao. The design configuration of a parabolic approximation bell nozzle
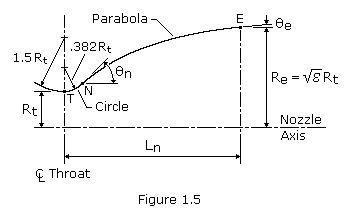
is shown in Figure 1.5. The nozzle contour immediately upstream of the
throat T is a circular arc with a radius of 1.5 Rt. The divergent section nozzle contour is made up of a circular entrance section with a radius of 0.382 Rt from the throat T to the point N and parabola from there to the exit E.
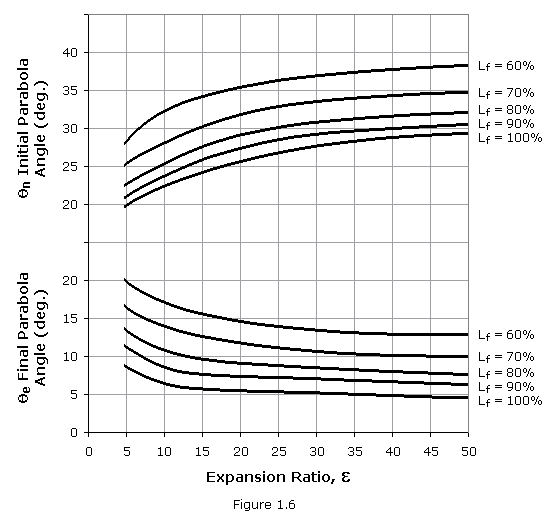
Design of a specific nozzle requires the following data: throat diameter Dt, axial length of the nozzle from throat to exit plane Ln (or the desired fractional length, Lf, based on a 15-degree conical nozzle), expansion ratio 
, initial wall angle of the parabola n, and nozzle exit wall angle e. The wall angles n and e
are shown in Figure 1.6 as a function of the expansion ratio. Optimum
nozzle contours can be approximated very accurately by selecting the
proper inputs. Although no allowance is made for different propellant
combinations, experience has shown only small effect of the specific
heat ratio upon the contour.
, initial wall angle of the parabola n, and nozzle exit wall angle e. The wall angles n and e
are shown in Figure 1.6 as a function of the expansion ratio. Optimum
nozzle contours can be approximated very accurately by selecting the
proper inputs. Although no allowance is made for different propellant
combinations, experience has shown only small effect of the specific
heat ratio upon the contour.
Combustion Chamber
The combustion chamber serves as an envelope to retain the
propellants for a sufficient period to ensure complete mixing and
combustion. The required stay time, or combustion residence time, is a
function of many parameters. The theoretically required combustion
chamber volume is a function of the mass flow rate of the propellants,
the average density of the combustion products, and the stay time needed
for efficient combustion. This relationship can be expressed by the
following equation:
where Vc is the chamber volume, q is the propellant mass flow rate, V is the average specific volume, and ts is the propellant stay-time.
A useful parameter relative to chamber volume and residence time is the characteristic length, L* (pronounced "L star"), the chamber volume divided by the nozzle sonic throat area:
The L* concept is much easier to visualize than the more
elusive "combustion residence time", expressed in small fractions of a
second. Since the value of At is in nearly direct proportion to the product of q and V, L* is essentially a function of ts.
The customary method of establishing the L* of a new
thrust chamber design largely relies on past experience with similar
propellants and engine size. Under a given set of operating conditions,
such as type of propellant, mixture ratio, chamber pressure, injector
design, and chamber geometry, the value of the minimum required L* can only be evaluated by actual firings of experimental thrust chambers. Typical L* values for various propellants are shown in the table below. With throat area and minimum required L* established, the chamber volume can be calculated by equation (1.33).
Table 1: Chamber Characteristic Length, L*
| Propellant Combination | L*, cm |
|---|---|
| Nitric acid/hydrazine-base fuel | 76-89 |
| Nitrogen tetroxide/hydrazine-base fuel | 76-89 |
| Hydrogen peroxide/RP-1 (including catalyst bed) | 152-178 |
| Liquid oxygen/RP-1 | 102-127 |
| Liquid oxygen/ammonia | 76-102 |
| Liquid oxygen/liquid hydrogen (GH2 injection) | 56-71 |
| Liquid oxygen/liquid hydrogen (LH2 injection) | 76-102 |
| Liquid fluorine/liquid hydrogen (GH2 injection) | 56-66 |
| Liquid fluorine/liquid hydrogen (LH2 injection) | 64-76 |
| Liquid fluorine/hydrazine | 61-71 |
| Chlorine trifluoride/hydrazine-base fuel | 51-89 |
Three geometrical shapes have been used in combustion chamber design -
spherical, near-spherical, and cylindrical - with the cylindrical
chamber being employed most frequently in the United States. Compared to
a cylindrical chamber of the same volume, a spherical or near-spherical
chamber offers the advantage of less cooling surface and weight;
however, the spherical chamber is more difficult to manufacture and has
provided poorer performance in other respects.
The total combustion process, from injection of the reactants
until completion of the chemical reactions and conversion of the
products into hot gases, requires finite amounts of time and volume, as
expressed by the characteristic length L*. The value of this factor is significantly greater than the linear length between injector face and throat plane. The contraction ratio
is defined as the major cross-sectional area of the combuster divided
by the throat area. Typically, large engines are constructed with a low
contraction ratio and a comparatively long length; and smaller chambers
employ a large contraction ratio with a shorter length, while still
providing sufficient L* for adequate vaporization and combustion dwell-time.
As a good place to start, the process of sizing a new combustion
chamber examines the dimensions of previously successful designs in the
same size class and plotting such data in a rational manner. The throat
size of a new engine can be generated with a fair degree of confidence,
so it makes sense to plot the data from historical sources in relation
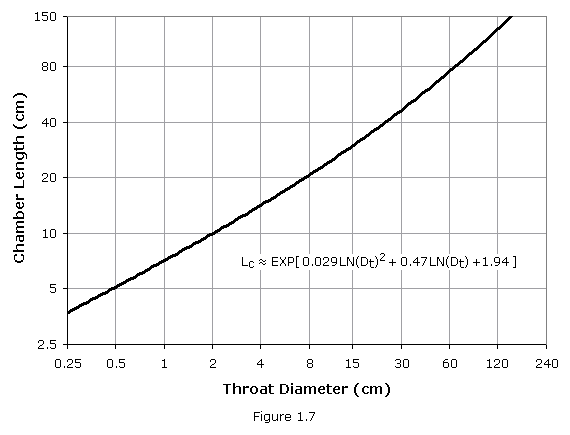
to throat diameter. Figure 1.7 plots chamber length as a function of
throat diameter (with approximating equation). It is important that the
output of any modeling program not be slavishly applied, but be
considered a logical starting point for specific engine sizing.
The basic elements of a cylindrical thrust-chamber are identified in
Figure 1.4. In design practice, it has been arbitrarily defined that the
combustion chamber volume includes the space between the injector face
and the nozzle throat plane. The approximate volume of the combustion
chamber can be expressed by the following equation:
Rearranging equation (1.34) we get the following, which can be solved for the chamber diameter via iteration:
Injector
The injector, as the name implies, injects the propellants into
the combustion chamber in the right proportions and the right conditions
to yield an efficient, stable combustion process. Placed at the
forward, or upper, end of the combustor, the injector also performs the
structural task of closing off the top of the combustion chamber against
the high pressure and temperature it contains. The injector has been
compared to the carburetor of an automobile engine, since it provides
the fuel and oxidizer at the proper rates and in the correct
proportions, this may be an appropriate comparison. However, the
injector, located directly over the high-pressure combustion, performs
many other functions related to the combustion and cooling processes and
is much more important to the function of the rocket engine than the
carburetor is for an automobile engine.
No other component of a rocket engine has as great an impact upon
engine performance as the injector. In various and different
applications, well-designed injectors may have a fairly wide spread in
combustion efficiency, and it is not uncommon for an injector with C*
efficiency as low as 92% to be considered acceptable. Small engines
designed for special purposes, such as attitude control, may be
optimized for response and light weight at the expense of combustion
efficiency, and may be deemed very satisfactory even if efficiency falls
below 90%. In general, however, recently well-designed injection
systems have demonstrated C* efficiencies so close to 100% of
theoretical that the ability to measure this parameter is the limiting
factor in its determination. High levels of combustion efficiency derive
from uniform distribution of the desired mixture ratio and fine
atomization of the liquid propellants. Local mixing within the
injection-element spray pattern must take place at virtually a
microscopic level to ensure combustion efficiencies approaching 100%.
Combustion stability is also a very important requirement for a
satisfactory injector design. Under certain conditions, shock and
detonation waves are generated by local disturbances in the chamber,
possibly caused by fluctuations in mixing or propellant flow. These may
trigger pressure oscillations that are amplified and maintained by the
combustion processes. Such high-amplitude waves - referred to as combustion instability
- produce high levels of vibration and heat flux that can be very
destructive. A major portion of the design and development effort
therefore concerns stable combustion. High performance can become
secondary if the injector is easily triggered into destructive
instability, and many of the injector parameters that provide high
performance appear to reduce the stability margin.
Liquid bipropellant rocket engines can be categorized according to
their power cycles, that is, how power is derived to feed propellants to
the main combustion chamber. Described below are some of the more
common types.
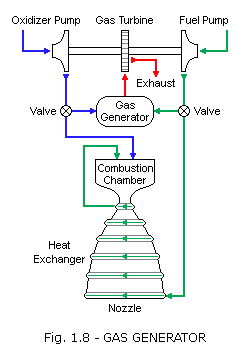
Gas-generator cycle: The gas-generator cycle, also called open cycle,
taps off a small amount of fuel and oxidizer from the main flow
(typically 2 to 7 percent) to feed a burner called a gas generator. The
hot gas from this generator passes through a turbine to generate power
for the pumps that send propellants to the combustion chamber. The hot
gas is then either dumped overboard or sent into the main nozzle
downstream. Increasing the flow of propellants into the gas generator
increases the speed of the turbine, which increases the flow of
propellants into the main combustion chamber, and hence, the amount of
thrust produced. The gas generator must burn propellants at a
less-than-optimal mixture ratio to keep the temperature low for the
turbine blades. Thus, the cycle is appropriate for moderate power
requirements but not high-power systems, which would have to divert a
large portion of the main flow to the less efficient gas-generator flow.
As in most rocket engines, some of the propellant in a gas
generator cycle is used to cool the nozzle and combustion chamber,
increasing efficiency and allowing higher engine temperature.
Staged combustion cycle: In a staged combustion cycle, also called closed cycle,
the propellants are burned in stages. Like the gas-generator cycle,
this cycle also has a burner, called a preburner, to generate gas for a
turbine. The preburner taps off and burns a small amount of one
propellant and a large amount of the other, producing an oxidizer-rich
or fuel-rich hot gas mixture that is mostly unburned vaporized
propellant. This hot gas is then passed through the turbine, injected
into the main chamber, and burned again with the remaining propellants.
The advantage over the gas-generator cycle is that all of the
propellants are burned at the optimal mixture ratio in the main chamber
and no flow is dumped overboard. The staged combustion cycle is often
used for high-power applications. The higher the chamber pressure, the
smaller and lighter the engine can be to produce the same thrust.
Development cost for this cycle is higher because the high pressures
complicate the development process. Further disadvantages are harsh
turbine conditions, high temperature piping required to carry hot gases,
and a very complicated feedback and control design.
Staged combustion was invented by Soviet engineers and first
appeared in 1960. In the West, the first laboratory staged combustion
test engine was built in Germany in 1963.
Expander cycle: The expander cycle is similar to
the staged combustion cycle but has no preburner. Heat in the cooling
jacket of the main combustion chamber serves to vaporize the fuel. The
fuel vapor is then passed through the turbine and injected into the main
chamber to burn with the oxidizer. This cycle works with fuels such as
hydrogen or methane, which have a low boiling point and can be vaporized
easily. As with the staged combustion cycle, all of the propellants are
burned at the optimal mixture ratio in the main chamber, and typically
no flow is dumped overboard; however, the heat transfer to the fuel
limits the power available to the turbine, making this cycle appropriate
for small to midsize engines. A variation of the system is the open, or
bleed, expander cycle, which uses only a portion of the fuel to drive
the turbine. In this variation, the turbine exhaust is dumped overboard
to ambient pressure to increase the turbine pressure ratio and power
output. This can achieve higher chamber pressures than the closed
expander cycle although at lower efficiency because of the overboard
flow.
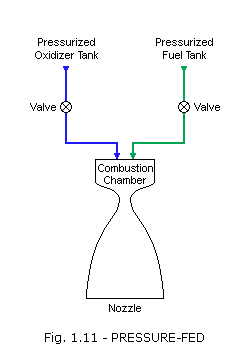
Pressure-fed cycle: The simplest system, the
pressure-fed cycle, does not have pumps or turbines but instead relies
on tank pressure to feed the propellants into the main chamber. In
practice, the cycle is limited to relatively low chamber pressures
because higher pressures make the vehicle tanks too heavy. The cycle can
be reliable, given its reduced part count and complexity compared with
other systems.
The heat created during combustion in a rocket engine is contained
within the exhaust gases. Most of this heat is expelled along with the
gas that contains it; however, heat is transferred to the thrust chamber
walls in quantities sufficient to require attention.
Thrust chamber designs are generally categorized or identified by
the hot gas wall cooling method or the configuration of the coolant
passages, where the coolant pressure inside may be as high as 500
atmospheres. The high combustion temperatures (2,500 to 3,600o K) and the high heat transfer rates (up to 16 kJ/cm2-s)
encountered in a combustion chamber present a formidable challenge to
the designer. To meet this challenge, several chamber cooling techniques
have been utilized successfully. Selection of the optimum cooling
method for a thrust chamber depends on many considerations, such as type
of propellant, chamber pressure, available coolant pressure, combustion
chamber configuration, and combustion chamber material.
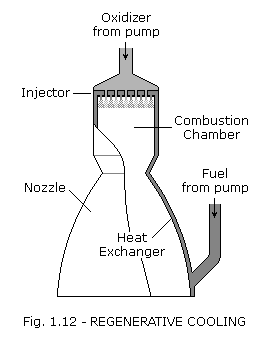
Regenerative cooling is the most widely used method of
cooling a thrust chamber and is accomplished by flowing high-velocity
coolant over the back side of the chamber hot gas wall to convectively
cool the hot gas liner. The coolant with the heat input from cooling the
liner is then discharged into the injector and utilized as a
propellant.
Earlier thrust chamber designs, such as the V-2 and Redstone, had
low chamber pressure, low heat flux and low coolant pressure
requirements, which could be satisfied by a simplified "double wall
chamber" design with regenerative and film cooling. For subsequent
rocket engine applications, however, chamber pressures were increased
and the cooling requirements became more difficult to satisfy. It became
necessary to design new coolant configurations that were more efficient
structurally and had improved heat transfer characteristics.
This led to the design of "tubular wall" thrust chambers, by far
the most widely used design approach for the vast majority of large
rocket engine applications. These chamber designs have been successfully
used for the Thor, Jupiter, Atlas, H-1, J-2, F-1, RS-27 and several
other Air Force and NASA rocket engine applications. The primary
advantage of the design is its light weight and the large experience
base that has accrued. But as chamber pressures and hot gas wall heat
fluxes have continued to increase (>100 atm), still more effective
methods have been needed.
One solution has been "channel wall" thrust chambers, so named
because the hot gas wall cooling is accomplished by flowing coolant
through rectangular channels, which are machined or formed into a hot
gas liner fabricated from a high-conductivity material, such as copper
or a copper alloy. A prime example of a channel wall combustion chamber
is the SSME, which operates at 204 atmospheres nominal chamber pressure
at 3,600 K for a duration of 520 seconds. Heat transfer and structural
characteristics are excellent.
In addition to the regeneratively cooled designs mentioned above,
other thrust chamber designs have been fabricated for rocket engines
using dump cooling, film cooling, transpiration cooling, ablative liners
and radiation cooling. Although regeneratively cooled combustion
chambers have proven to be the best approach for cooling large liquid
rocket engines, other methods of cooling have also been successfully
used for cooling thrust chamber assemblies. Examples include:
Dump cooling, which is similar to regenerative
cooling because the coolant flows through small passages over the back
side of the thrust chamber wall. The difference, however, is that after
cooling the thrust chamber, the coolant is discharged overboard through
openings at the aft end of the divergent nozzle. This method has limited
application because of the performance loss resulting from dumping the
coolant overboard. To date, dump cooling has not been used in an actual
application.
Film cooling provides protection from excessive
heat by introducing a thin film of coolant or propellant through
orifices around the injector periphery or through manifolded orifices in
the chamber wall near the injector or chamber throat region. This
method is typically used in high heat flux regions and in combination
with regenerative cooling.
Transpiration cooling provides coolant (either
gaseous or liquid propellant) through a porous chamber wall at a rate
sufficient to maintain the chamber hot gas wall to the desired
temperature. The technique is really a special case of film cooling.
With ablative cooling, combustion gas-side wall
material is sacrificed by melting, vaporization and chemical changes to
dissipate heat. As a result, relatively cool gases flow over the wall
surface, thus lowering the boundary-layer temperature and assisting the
cooling process.
With radiation cooling, heat is radiated from the
outer surface of the combustion chamber or nozzle extension wall.
Radiation cooling is typically used for small thrust chambers with a
high-temperature wall material (refractory) and in low-heat flux
regions, such as a nozzle extension.
Solid rockets motors store propellants in solid form. The fuel is
typically powdered aluminum and the oxidizer is ammonium perchlorate. A
synthetic rubber binder such as polybutadiene holds the fuel and
oxidizer powders together. Though lower performing than liquid
propellant rockets, the operational simplicity of a solid rocket motor
often makes it the propulsion system of choice.
Solid Fuel Geometry
A solid fuel's geometry determines the area and contours of its
exposed surfaces, and thus its burn pattern. There are two main types of
solid fuel blocks used in the space industry. These are cylindrical
blocks, with combustion at a front, or surface, and cylindrical blocks
with internal combustion. In the first case, the front of the flame
travels in layers from the nozzle end of the block towards the top of
the casing. This so-called end burner produces constant thrust
throughout the burn. In the second, more usual case, the combustion
surface develops along the length of a central channel. Sometimes the
channel has a star shaped, or other, geometry to moderate the growth of
this surface.
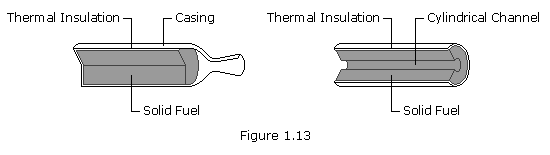
The shape of the fuel block for a rocket is chosen for the particular
type of mission it will perform. Since the combustion of the block
progresses from its free surface, as this surface grows, geometrical
considerations determine whether the thrust increases, decreases or
stays constant.
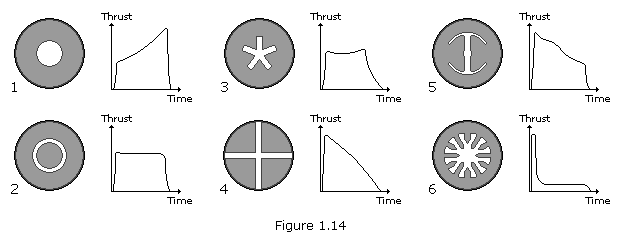
Fuel blocks with a cylindrical channel (1) develop their thrust
progressively. Those with a channel and also a central cylinder of fuel
(2) produce a relatively constant thrust, which reduces to zero very
quickly when the fuel is used up. The five pointed star profile (3)
develops a relatively constant thrust which decreases slowly to zero as
the last of the fuel is consumed. The 'cruciform' profile (4) produces
progressively less thrust. Fuel in a block with a 'double anchor'
profile (5) produces a decreasing thrust which drops off quickly near
the end of the burn. The 'cog' profile (6) produces a strong inital
thrust, followed by an almost constant lower thrust.
Burn Rate
The burning surface of a rocket propellant grain recedes in a
direction perpendicular to this burning surface. The rate of regression,
typically measured in millimeters per second (or inches per second), is
termed burn rate. This rate can differ significantly for
different propellants, or for one particular propellant, depending on
various operating conditions as well as formulation. Knowing
quantitatively the burning rate of a propellant, and how it changes
under various conditions, is of fundamental importance in the successful
design of a solid rocket motor.
Propellant burning rate is influenced by certain factors, the
most significant being: combustion chamber pressure, initial temperature
of the propellant grain, velocity of the combustion gases flowing
parallel to the burning surface, local static pressure, and motor
acceleration and spin. These factors are discussed below.
- Burn rate is profoundly affected by chamber pressure. The usual representation of the pressure dependence on burn rate is the Saint-Robert's Law,
where r is the burn rate, a is the burn rate coefficient, n is the pressure exponent, and Pc is the combustion chamber pressure. The values of a and n are determined empirically for a particular propellant formulation and cannot be theoretically predicted. It is important to realize that a single set of a, n values are typically valid over a distinct pressure range. More than one set may be necessary to accurately represent the full pressure regime of interest.Example a, n values are 5.6059* (pressure in MPa, burn rate in mm/s) and 0.35 respectively for the Space Shuttle SRBs, which gives a burn rate of 9.34 mm/s at the average chamber pressure of 4.3 MPa.* NASA publications gives a burn rate coefficient of 0.0386625 (pressure in PSI, burn rate in inch/s). - Temperature affects the rate of chemical reactions and thus the initial temperature of the propellant grain influences burning rate. If a particular propellant shows significant sensitivity to initial grain temperature, operation at temperature extremes will affect the time-thrust profile of the motor. This is a factor to consider for winter launches, for example, when the grain temperature may be lower than "normal" launch conditions.
- For most propellants, certain levels of local combustion gas velocity (or mass flux) flowing parallel to the burning surface leads to an increased burning rate. This "augmentation" of burn rate is referred to as erosive burning, with the extent varying with propellant type and chamber pressure. For many propellants, a threshold flow velocity exists. Below this flow level, either no augmentation occurs, or a decrease in burn rate is experienced (negative erosive burning).The effects of erosive burning can be minimized by designing the motor with a sufficiently large port-to-throat area ratio (Aport/At). The port area is the cross-section area of the flow channel in a motor. For a hollow-cylindrical grain, this is the cross-section area of the core. As a rule of thumb, the ratio should be a minimum of 2 for a grain L/D ratio of 6. A greater Aport/At ratio should be used for grains with larger L/D ratios.
- In an operating rocket motor, there is a pressure drop along the axis of the combustion chamber, a drop that is physically necessary to accelerate the increasing mass flow of combustion products toward the nozzle. The static pressure is greatest where gas flow is zero, that is, at the front of the motor. Since burn rate is dependant upon the local pressure, the rate should be greatest at this location. However, this effect is relatively minor and is usually offset by the counter-effect of erosive burning.
- Burning rate is enhanced by acceleration of the motor. Whether the acceleration is a result of longitudinal force (e.g. thrust) or spin, burning surfaces that form an angle of about 60-90o with the acceleration vector are prone to increased burn rate.
It is sometimes desirable to modify the burning rate such that it is
more suitable to a certain grain configuration. For example, if one
wished to design an end burner grain, which has a relatively small
burning area, it is necessary to have a fast burning propellant. In
other circumstances, a reduced burning rate may be sought after. For
example, a motor may have a large L/D ratio to generate sufficiently
high thrust, or it may be necessary for a particular design to restrict
the diameter of the motor. The web would be consequently thin, resulting
in short burn duration. Reducing the burning rate would be beneficial.
There are a number of ways of modifying the burning rate:
decrease the oxidizer particle size, increase or reduce the percentage
of oxidizer, adding a burn rate catalyst or suppressant, and operate the
motor at a lower or higher chamber pressure. These factors are
discussed below.
- The effect of the oxidizer particle size on burn rate seems to be influenced by the type of oxidizer. Propellants that use ammonium perchlorate (AP) as the oxidizer have a burn rate that is significantly affected by AP particle size. This most likely results from the decomposition of AP being the rate-determining step in the combustion process.
- The burn rate of most propellants is strongly influenced by the oxidizer/fuel ratio. Unfortunately, modifying the burn rate by this means is quite restrictive, as the performance of the propellant, as well as mechanical properties, are also greatly affected by the O/F ratio.
- Certainly the best and most effective means of increasing the burn rate is the addition of a catalyst to the propellant mixture. A catalyst is a chemical compound that is added in small quantities for the sole purpose of tailoring the burning rate. A burn rate suppressant is an additive that has the opposite effect to that of a catalyst – it is used to decrease the burn rate.
- For a propellant that follows the Saint-Robert's burn rate law, designing a rocket motor to operate at a lower chamber pressure will provide for a lower burning rate. Due to the nonlinearity of the pressure-burn rate relationship, it may be necessary to significantly reduce the operating pressure to get the desired burning rate. The obvious drawback is reduced motor performance, as specific impulse similarly decays with reducing chamber pressure.
Product Generation Rate
The rate at which combustion products are generated is expressed
in terms of the regression speed of the grain. The product generation
rate integrated over the port surface area is
where q is the combustion product generation rate at the propellant surface, 
p is the solid propellant density, Ab is the area of the burning surface, and r is the propellant burn rate.
p is the solid propellant density, Ab is the area of the burning surface, and r is the propellant burn rate.
If the propellant density is unknown, it can be derived from the mass
fraction and density of the individual constituents, as follows:
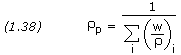
where w is the mass fraction and the subscript i denotes the individual constituents. This is the ideal density; the actual
density is typically 94%-97% of the ideal density, owing to tiny voids
in the grain, and is dependant upon the manufacturing technique.
Condensed-Phase Mass
It is important to note that the combustion products may consist
of both gaseous and condensed-phase mass. The condensed-phase, which
manifests itself as smoke, may be either solid or liquid particles. Only
the gaseous products contribute to pressure development. The
condensed-phase certainly does, however, contribute to the thrust of the
rocket motor, due to its mass and velocity.
The occurrence of solids or liquids in a rocket's exhaust leads to a reduction in performance for a number of reasons:
- This portion of the combustion mass cannot perform any expansion work and, therefore, does not contribute to acceleration of the exhaust flow.
- The higher effective molecular weight of these products lowers the characteristic exhaust velocity, C*.
- Due to thermal inertia, the heat of the condensed species is partly ejected out of the nozzle before transferring this heat to the surrounding gas, and is, therefore, not converted to kinetic energy. This is known as particle thermal lag.
- Likewise, due to the relatively large mass of the particles (compared to the gases), these cannot accelerate as rapidly as the surrounding gases, especially in that portion of the nozzle where flow acceleration is extremely high (throat region). Acceleration of the particles depends upon frictional drag in the gas flow, which necessitates a differential velocity. The net result is that the condensed-phase particles exit the nozzle at a lower velocity than the gases. This is referred to as particle velocity lag.
Chamber Pressure
The pressure curve of a rocket motor exhibits transient and
steady state behavior. The transient phases are when the pressure varies
substantially with time – during the ignition and start-up phase, and
following complete (or nearly complete) grain consumption when the
pressure falls down to ambient level during the tail-off phase. The
variation of chamber pressure during the steady state burning phase is
due mainly to variation of grain geometry with associated burn rate
variation. Other factors may play a role, however, such as nozzle throat
erosion and erosive burn rate augmentation.
By far the most widely used type of propulsion for spacecraft
attitude and velocity control is monopropellant hydrazine. Its excellent
handling characteristics, relative stability under normal storage
conditions, and clean decomposition products have made it the standard.
The general sequence of operations in a hydrazine thruster is:
- When the attitude control system signals for thruster operation, an electric solenoid valve opens allowing hydrazine to flow. The action may be pulsed (as short as 5 ms) or long duration (steady state).
- The pressure in the propellant tank forces liquid hydrazine into the injector. It enters as a spray into the thrust chamber and contacts the catalyst beds.
- The catalyst bed consists of alumina pellets impregnated with iridium. Incoming hydrazine heats to its vaporizing point by contact with the catalyst bed and with the hot gases leaving the catalyst particles. The temperature of the hydrazine rises to a point where the rate of its decomposition becomes so high that the chemical reactions are self-sustaining.
- By controlling the flow variables and the geometry of the catalyst chamber, a designer can tailor the proportion of chemical products, the exhaust temperature, the molecular weight, and thus the enthalpy for a given application. For a thruster application where specific impulse is paramount, the designer attempts to provide 30-40% ammonia dissociation, which is about the lowest percentage that can be maintained reliably. For gas-generator application, where lower temperature gases are usually desired, the designer provides for higher levels of ammonia dissociation.
- Finally, in a space thruster, the hydrazine decomposition products leave the catalyst bed and exit from the chamber through a high expansion ratio exhaust nozzle to produce thrust.
Monopropellant hydrazine thrusters typically produce a specific impulse of about 230 to 240 seconds.
Other suitable propellants for catalytic decomposition engines
are hydrogen peroxide and nitrous oxide, however the performance is
considerably lower than that obtained with hydrazine - specific impulse
of about 150 s with H2O2 and about 170 s with N2O.
Monopropellant systems have successfully provided orbit
maintenance and attitude control functions, but lack the performance to
provide weight-efficient large V
maneuvers required for orbit insertion. Bipropellant systems are
attractive because they can provide all three functions with one higher
performance system, but they are more complex than the common solid
rocket and monopropellant combined systems. A third alternative are dual mode
systems. These systems are hybrid designs that use hydrazine both as a
fuel for high performance bipropellant engines and as a monopropellant
with conventional low-thrust catalytic thrusters. The hydrazine is fed
to both the bipropellant engines and the monopropellant thrusters from a
common fuel tank.
V
maneuvers required for orbit insertion. Bipropellant systems are
attractive because they can provide all three functions with one higher
performance system, but they are more complex than the common solid
rocket and monopropellant combined systems. A third alternative are dual mode
systems. These systems are hybrid designs that use hydrazine both as a
fuel for high performance bipropellant engines and as a monopropellant
with conventional low-thrust catalytic thrusters. The hydrazine is fed
to both the bipropellant engines and the monopropellant thrusters from a
common fuel tank.
Cold gas propulsion is just a controlled, pressurized gas
source and a nozzle. It represents the simplest form of rocket engine.
Cold gas has many applications where simplicity and/or the need to avoid
hot gases are more important than high performance. The Manned
Maneuvering Unit used by astronauts is an example of such a system.
Multistage rockets allow improved payload capability for vehicles with a high V
requirement such as launch vehicles or interplanetary spacecraft. In a
multistage rocket, propellant is stored in smaller, separate tanks
rather than a larger single tank as in a single-stage rocket. Since each
tank is discarded when empty, energy is not expended to accelerate the
empty tanks, so a higher total V is obtained. Alternatively, a larger payload mass can be accelerated to the same total V. For convenience, the separate tanks are usually bundled with their own engines, with each discardable unit called a stage.
V
requirement such as launch vehicles or interplanetary spacecraft. In a
multistage rocket, propellant is stored in smaller, separate tanks
rather than a larger single tank as in a single-stage rocket. Since each
tank is discarded when empty, energy is not expended to accelerate the
empty tanks, so a higher total V is obtained. Alternatively, a larger payload mass can be accelerated to the same total V. For convenience, the separate tanks are usually bundled with their own engines, with each discardable unit called a stage.
Multistage rocket performance is described by the same rocket
equation as single-stage rockets, but must be determined on a
stage-by-stage basis. The velocity increment, Vi, for each stage is calculated as before,
Vi, for each stage is calculated as before,
where moi represents the total vehicle mass when stage i is ignited, and mfi is the total vehicle mass when stage i is burned out but not yet discarded.
It is important to realize that the payload mass for any stage consists
of the mass of all subsequent stages plus the ultimate payload itself.
The velocity increment for the vehicle is then the sum of those for the
individual stages where n is the total number of stages.
We define the payload fraction as the ratio of payload mass to initial mass, or mpl/mo.
For a multistage vehicle with dissimilar stages, the overall vehicle payload fraction depends on how the V requirement is partitioned among stages. Payload fractions will be reduced if the V is partitioned suboptimally. The optimal distribution may be determined by trial and error. A V distribution is postulated and the resulting payload fraction calculated. The V distribution is varied until the payload fraction is maximized. Once the V
distribution is selected, vehicle sizing is accomplished by starting
with the uppermost or final stage (whose payload is the actual
deliverable payload) and calculating the initial mass of this assembly.
This assembly then forms the payload for the previous stage and the
process repeats until all stages are sized. Results reveal that to
maximize payload fraction for a given V requirement:
V requirement is partitioned among stages. Payload fractions will be reduced if the V is partitioned suboptimally. The optimal distribution may be determined by trial and error. A V distribution is postulated and the resulting payload fraction calculated. The V distribution is varied until the payload fraction is maximized. Once the V
distribution is selected, vehicle sizing is accomplished by starting
with the uppermost or final stage (whose payload is the actual
deliverable payload) and calculating the initial mass of this assembly.
This assembly then forms the payload for the previous stage and the
process repeats until all stages are sized. Results reveal that to
maximize payload fraction for a given V requirement:
1. Stages with higher Isp should be above stages with lower Isp.
2. More V should be provided by the stages with the higher Isp.
V should be provided by the stages with the higher Isp.
3. Each succeeding stage should be smaller than its predecessor.
4. Similar stages should provide the same V.
V.
Compiled, edited and written in part by Robert A. Braeunig, 1997, 2005, 2007, 2009, 2012.
Tidak ada komentar:
Posting Komentar
ORANMG PINTAR UNTUK TAMBAH PENGETAHUAN PASTI BACA BLOG 'ROTE PINTAR'. TERNYATA 15 NEGARA ASING JUGA SENANG MEMBACA BLOG 'ROTE PINTAR' TERIMA KASIG KEPADA SEMUA PEMBACA BLOG 'ROTE PINTAR' DIMANA SAJA, KAPAN SAJA DAN OLEG SIAPA SAJA. NAMUN SAYA MOHON MAAF KARENA DALAM BEBERAPA HALAMAN DARI TIAP JUDUL TERDAPAT SAMBUNGAN KATA YANG KURANG SEMPURNA PADA SISI PALING KANAN DARI SETIAP HALAM TIDAK BERSAMBUNG BAIK SUKU KATANYA, OLEH KARENA ADA TERDAPAT EROR DI KOMPUTER SAAT MEMASUKKAN DATANYA KE BLOG SEHINGGA SEDIKIT TERGANGGU, DAN SAYA SENDIRI BELUM BISA MENGATASI EROR TERSEBUT, SEHINGGA PARA PEMBACA HARAP MAKLUM, NAMUN DIHARAPKAN BISA DAPAT MEMAHAMI PENGERTIANNYA SECARA UTUH. SEKALI LAGI MOHON MAAF DAN TERIMA KASIH BUAT SEMUA PEMBACA BLOG ROTE PINTAR, KIRANYA DATA-DATA BARU TERUS MENAMBAH ISI BLOG ROTE PINTAR SELANJUTNYA. DARI SAYA : Drs.Simon Arnold Julian Jacob-- Alamat : Jln.Jambon I/414J- Rt.10 - Rw.03 - KRICAK - JATIMULYO - JOGJAKARTA--INDONESIA-- HP.082135680644 - Email : saj_jacob1940@yahoo.co.id.com BLOG ROTE PINTAR : sajjacob.blogspot.com TERIMA KASIH BUAT SEMUA.